Sturnira perla, V, Pablo Jarrín - & Kunz, Thomas H., 2011

V, Pablo Jarrín - & Kunz, Thomas H., 2011, A new species of Sturnira (Chiroptera: Phyllostomidae) from the Choco forest of Ecuador, Zootaxa 2755, pp. 1-35 : 6-18

Sturnira perla , new species

Figure 2

Holotype. QCAZ 120, adult female with skin and skull (S/S). The skull is in excellent condition, while the skin is slightly damaged. Collected on 26 June 1990, by Felipe Campos. Ecuador, Provincia Santo Domingo de los Tsachilas, Bosque Protector La Perla at 200 meters of altitude (0º08'N, 79º30'W), 2 km south of La Concordia (locality # 5 in Fig. 3 ).

Paratypes. Six additional specimens are from Bosque Protector La Perla (locality # 5 in Fig. 3 ), collected on 30 April 1992 by Felipe Campos, except when mentioned: QCAZ 119, pregnant (S/S), 28 April 1990; QCAZ 437, possibly lactating, alcohol and skull (A/S), 30 April 1992; QCAZ 438, adult male (A/S); QCAZ 439, adult male (A/S); QCAZ 440, adult female (A/S); QCAZ 542 (QLP-061), possibly lactating, 28 June 1990 (A/S). Three specimens from Ecuador, Provincia Esmeraldas, Borbón, Río Santiago, Estero María, comuna Selva Alegre at 50 meters of altitude (1º06'N 78º59'W), collected on 24 October 1996 by Nestor Acosta and Lincoln Nolivos: QCAZ 1909, adult female (A/S); QCAZ 1912, adult female (A/S); QCAZ 1920 adult male with scrotal testes (A/S) (locality # 1 in Fig. 3 ). One specimen from Provincia Esmeraldas, Borbón, Río Cayapas Angostura at 35 m (0º53'14''N, 78º50'38''W), collected on 24 October 1996 by unknown collector: QCAZ 2076, adult female (A/S) (locality # 2 in Fig. 3 ). One specimen from Ecuador, Provincia Esmeraldas, Reserva La Mayronga, Sector ATP, en el bosque al borde del estero at 80 m (80 m), collected on 26 October 1998 by Igor Castro and Lincoln Nolivos: MECN 1665, adult male with scrotal testes (S/S) (locality # 4 in Fig. 3 ).

Other specimens. Additionally, Iudica (2000) mentions two specimens that were “made available” to him “through the courtesy of Timothy J. McCarthy and Luis Albuja V.”, one of which is included in this study as part of the molecular analysis ( CAI 226). These two specimens are from Ecuador, Provincia Esmeraldas, “Near Nueva Vida, 1.9 km N, 10.4 km E Codesa-Sade compound at Río Esmeraldas, Manzano at 450-460 m (0º32'N, 79º17'W)”: CMNH 112823 ( CAI 181), pregnant, 11 December 1991 (A/S); CMNH 112822 ( CAI 226), male, 8 December 1991 (S/S) (locality # 3 in Fig. 3 ).

Etymology. Perla is a word with deep Latin roots having the same meaning as pearl in modern English ( Segura 2006). Here the term perla is used as a noun in apposition. Figuratively, perla means something very precious. It is also a metaphor to the globular shape of the skull of this species and honors the Bosque Protector La Perla , the locality where the majority of samples were found.

Distribution. Known only from the tropical rainforest lowlands of the Choco forests in Ecuador ( Fig. 3 , Appendix 1). Its presence may extend to the Colombian Choco. Sturnira perla has been found near the coastal plain (35 m) and up to 220 m. This is the lowest elevational range registered for any other species of Sturnira in Ecuador, with sympatric species reaching higher altitudes up to 1618 m for S. lilium, 1600 m for S. luisi and 1450 m for S. tildae .

n=207. Data is presented as: mean [95% confidence interval around the mean] (minimum-maximum) standard deviation. Two

characters present no overlap in their means between Sturnira perla and the other species, and are highlighted by an ending

asterisk. All characters are in millimeters except for ACM which is an angle in degrees. Sample size is Sturnira lilium =103, S.

luisi =76, S. perla =12 and S. tildae =15.

continued next page

Diagnosis. Sturnira perla was previously recognized as an independent phylogenetic lineage by Iudica (2000). The globular shape of the skull is remarkably distinct, not only in terms of Euclidean distances in morphospace as is explained below, but also to the point of being distinguished by simple visual inspection ( Fig. 2 ). The spherical skull is, in part, the consequence of an extremely blunt rostrum, robust and curved zygomatic arches and globular braincase. Also, the M3W is remarkably larger in this species against every other individual comparison.

Comparisons. Except for the overall architecture of the skull ( Fig. 2 ), Sturnira perla is externally cryptic, relative to the sympatric species S. lilum , S. luisi and S. tildae . Together, with other lowland species, S. perla shares the “lingual ridge of lower molars with no vertical division” (de la Torre 1961), and therefore is distinguished by this discrete trait from other species in the highlands, such as S. oporaphilum , S. bogotensis , S. erythromos , S. ludovici , and S. koopmanhilli . In this same sense, the presence of four lower incisors distinguishes S. perla from S. bidens and S. nana , both with two lower incisors. Also, and relative to other similar species, the third upper molar (M3) is remarkably large ( Table 1 ). Thus, S. perla can only be recognized by an inspection of the skinned skull and the quantification of its overall geometric structure. No other morphological characters, as are currently explained in the available literature, show convincing evidence of this species being distinct from other similar and closely related congeners. As it was explained earlier, the verbal and qualitative description of character states inherently relies on typological essences, with no information on distributional properties among species (sensu Mayr 1959; Jarrín-V. & Kunz 2008). Thus, the qualitative description of characters related to the facial structure (i.e. vibrissae, noseleaf, warts, etc.), length of hair, number of bands on dorsal and ventral hairs, etc., are all irrelevant or dubious characters for the diagnosis of species in this particular case. Perhaps, subsequent studies that consider the statistical distribution of these characters within a quantitative perspective may validate their usefulness for diagnosing species.

TABLE 2. Component loadings of a principal component analysis based on the covariance matrix of the log e -transformed linear characters for adult specimens of the Sturnira lilium-luisi-tildae complex in Ecuador (n = 279). A total of five components where extracted based on the average item variance for an eigenvalue> 1. The table includes the rescaled factor loadings for each variable, and the eigenvalues and proportion of variance explained by each component at the end of each column. Variables are ordered based on their highest loadings for PC I. Sample size is Sturnira lilium =103, S. luisi =76, S. perla =12 and S. tildae =15.

continued next page Eigenvalue 13 2.14 1.45 2.10 1.05 % of variation 48.27 7.94 5.36 7.78 3.881

Dental formula. (i 2 /2, c 1/1, p 2/2; m 3/3) x 2 = total 32. This is the same as most species in Sturnira , except for S. bidens and S. nana .

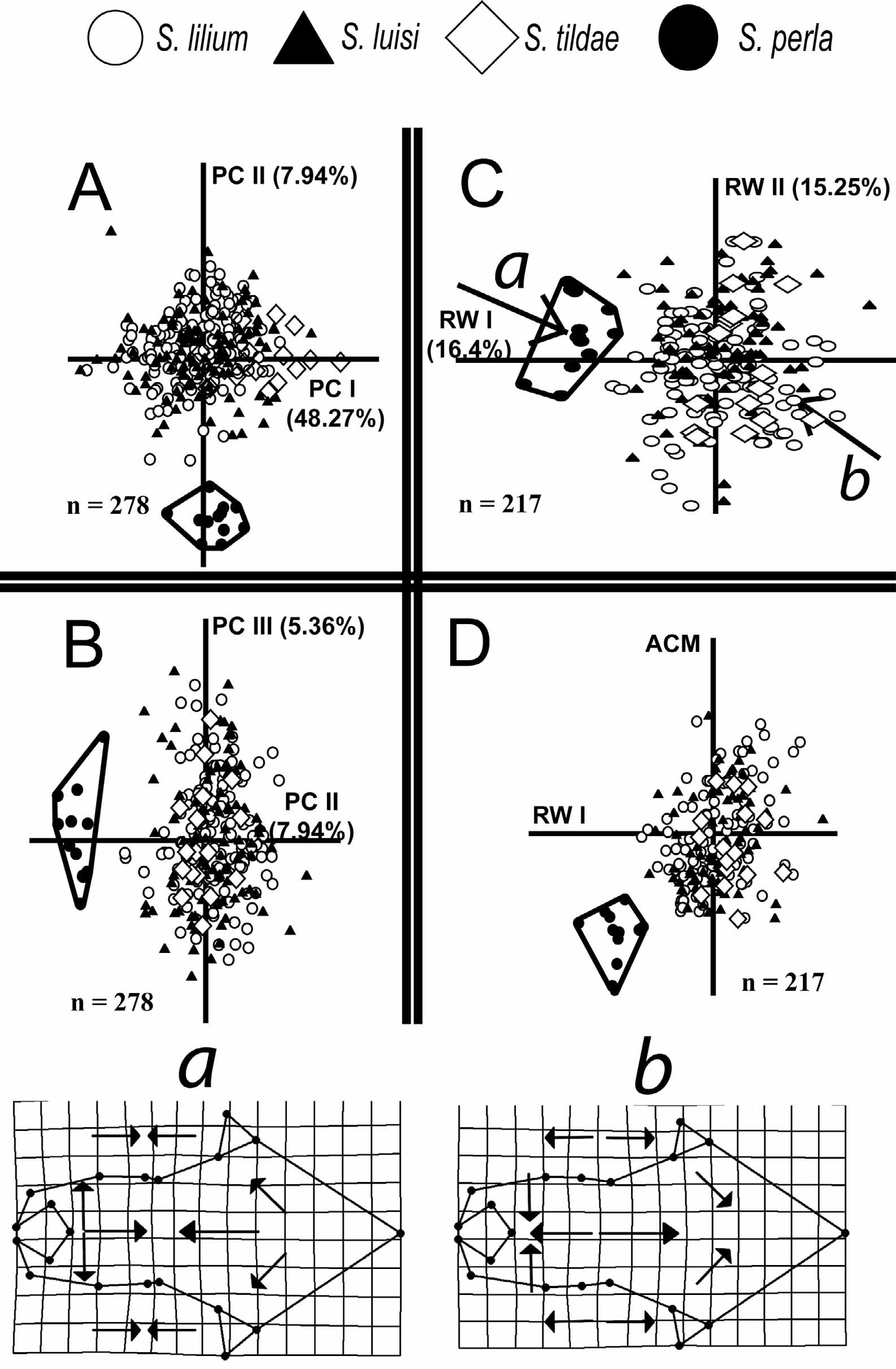
Statistical assessment of morphological variation. Overall size appears as an indistinct trait among lowland species, as can be observed from the frequency distributions for FA and GLS ( Fig. 4 ). The observed distribution for both traits approaches a normal curve (GLS: K-S = 0.03, df = 278, P> 0.2; FA: K-S = 0.05, df = 278, P = 0.09). This suggests a single statistically normal population; or otherwise, largely overlapping size variation among populations or species (i.e. Sturnira lilium , S. luisi , and S. tildae ). A simple inspection on the central tendency and dispersion estimates among species, further suggests that sharp boundaries occur exclusively for the character states of ACM and M3W in S. perla , especially if we consider the confidence interval around the mean ( Table 1 ). In a similar sense, there are no discernable or separate groups in the first two PCs, except for a noticeable boundary with consistently low values for PC II ( Fig. 5 A–B). High values in PC II represent mostly low values in M3W, as shown for loading indexes (Table 2). It is important to highlight that there is total overlap along PC I and PC III for S. perla in relation to its congeners. Thus, the differentiation based on this particular approach is one-dimensional. The other components above the unity eigenvalue completely overlap and are of minor comparative interest.

MANOVA FOR PC I–PC V and ACM p -value Effect size (ƛ2) Equality of covariance Box's M=208.11, F=1.26, df=126/3067.66 0.03

Joint centroids (MANOVA) Species - Pillai's trace=0.96, F=15.36, df=18/585 <0.001 0.32 Sex - Pillai's trace=0.16, F=6.19, df=6/193 <0.001 0.16 Species X Sex - Pillai's trace=0.52, F=0.58, df=18/585 0.92 0.02

Centroids (ANOVA) ACM on Species - F=14.93 <0.001 0.21 PC I on Species - F=15.06 <0.001 0.17 PC II on Species - F=73.34 <0.001 0.55 PC III on Species - F=0.41 0.81 0.01 PC IV on Species - F=11.2 <0.001 0.15 PC V on Species - F=8.05 <0.001 0.11 ACM on Sex - F=2.58 0.11 0.01 PC I on Sex - F=9.7 <0.01 0.05 PC II on Sex - F=3.86 0.18 0.01 PC III on Sex - F=2.92 0.07 0.02 PC IV on Sex - F=0.33 0.47 <0.01 PC V on Sex - F=6.25 <0.01 0.03 Marginal means (Bonferroni) for ACM S. perla vs S. lilium <0.001 S . perla vs. S. luisi <0.001 S . perla vs. S. tildae <0.001 Marginal means (Bonferroni) for PC II S. perla vs S. lilium <0.001 S . perla vs. S. luisi <0.001 S . perla vs. S. tildae <0.001 Marginal means (Bonferroni) for PC I S. tildae vs. S. perla <0.001 S . tildae vs. S. lilium <0.001 S . tildae vs. S. luisi <0.01 On the whole, the MANOVA points towards PC II as the factor responsible for the largest differences among species, with about half of the observed differences provided by this factor (η 2 = 0.55). No other factor shows a large effect in the MANOVA model ( Table 3 ). Sexual differences are constant throughout, but with small effect size (i.e. interaction term with P = 0.92 and η 2 <0.02). Based on the MANOVA, both ACM and PC II are responsible for the differences between Sturnira perla and the other species. In contrast to all other species, differences are also found for S. tildae along PC I; however, the differences along PC I are of minor impact relative to those attributable to PC II (η 2 = 0.17 vs. η 2 = 0.55, respectively) ( Table 3 ). Overall, the MANOVA shows equal error variances for all response variables, except for PC I. The model does not comply with the requisite for equality of covariance among groups. We assume that the lack of homogeneity of variance-covariance matrices does not represent a serious violation of the assumptions of MANOVA, being this GLS a relatively robust model ( Stevens 2002).

Boundaries are also present in the space spanned by the first two components of shape (i.e. RW 1 and RW 2), a demarcation that is also one-dimensional along RW 1 ( Fig. 5 C). Estimates of geometric deformation along the space depicted in Fig. 5 C conform to the overall pattern of variation that makes Sturnira perla a clearly distinct shape. Along this space, skulls in the region delineated as S. perla tend to experience an expansion of the braincase, a shortening of the zygomatic region, and a widening of the rostrum, giving other species a comparatively more slender appearance ( Fig. 5 a-b). The clearest separation is, nevertheless, obtained in the space spanned by RW 1 and ACM, with a bidimensional demarcation along both characters. The lower corner of quadrant III in this plot is for skulls showing a combination of wide angles for ACM and the characteristic round skull as previously noted ( Fig. 5 D). Accordingly, contrast tests on the position of centroids for the space in Figs. 5 C and 5D clearly indicate that it is only for S. perla where the observed differences are hardly due to chance. It is worth noting here that each model is robust (i.e. equal covariance matrices and error variance) and there are no differences in variation for shape between males and females for a given species ( Table 4 ).

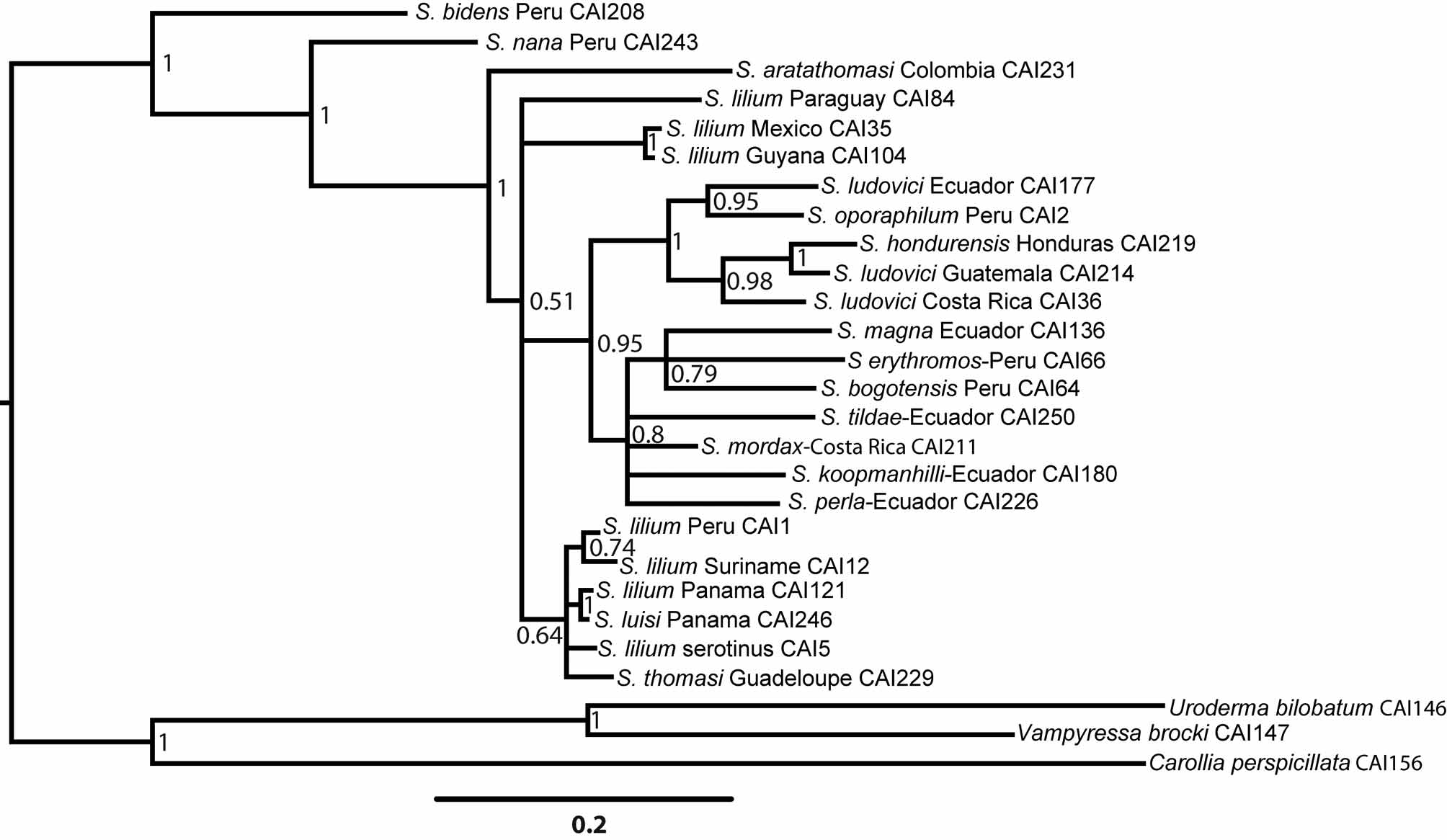
Genetic distances and phylogenetic relationships. The matrix of distances according to the K80 model and the GTR+I+Γ is found in Appendix 4, where the interpretation of the overall pattern is facilitated by color-coded cells. Both matrices are similar, with the same patterns of distance among taxa. For the case of K80 distances, the general pattern is for uniformity among all the ingroup species (x¯ =8.0%, sd =0.04, n=648), except for relatively low distances (0.05% - 2.5%) among the group of Sturnira lilium and S. luisi (CAI246, 229, 146, 121, 104, 12, 5, 1) and between the Central American S. hondurensis Goodwin (CAI219) and S. ludovici (CAI214). The largest average distance is for S. bidens from Peru (CAI208) (x¯ =11%, sd =0.03, n=27). Within the ingroup, S. perla maintains an average genetic distance of 7.1% (sd =0.03, n=27) relative to all other congeneric species ( Fig. 6 ); hence, it remains clearly distinct from the lowland group of S. lilium and S. luisi .

It is remarkable that Sturnira perla seems to share more recent common ancestry with the highland group of species (e.g. S. ludovici , S. oporaphilum , S. erythromos and S. bogotensis ) —including other typically lowland taxa as S. tildae and S. magna — rather than with emblematic and common species occurring at low elevations as are S. lilium and S. luisi ( Fig. 7 ). The other lowland species (e.g. S. lilum and S. luisi ) are related to S. perla through deeper nodes.The phylogenetic hypothesis presented here represents the one with the highest degree of resolution to be published so far.

Our contribution to understand phylogenetic relationships among Sturnira , although not central to the discussion of a new species, must be commented within the context of past phylogenetic propositions, which include all or most species of Sturnira . Owen (1987) used external body and cranial characters in an attempt to define the phenetic and phylogenetic relationships among species in the subfamily Stenodermatinae ; his consensus tree did not resolve the relationships among most of the species in Sturnira ( Fig 8 A). The analysis by Pacheco and Patterson (1991) was the first phylogenetic assessment made exclusively for Sturnira ( Fig. 8 B). Their approach recognized both S. oporaphilum and S. bogotensis as different taxa and sister to S. ludovici and S. erythromos respectively. The other definable lineage was a polytomy formed by S. lilium , S. luisi and S. thomasi . A close relationship between S. lilium and S. luisi , on both morphological and genetic grounds was recognized, also reporting the presence of an undescribed species endemic to Ecuador, named as S. sp. A and currently known as S. koopmanhilli ( McCarthy et al. 2006) . More recently, Iudica (2000), in his unpublished thesis, defined 17 species based on a partial sequence of the cytochrome b gene ( Fig. 8 C). Iudica’s work redefines S. parvidens and S. hondurensis as valid species. This phylogenetic analysis reinforced the hypotheses suggested previously by Pacheco and Patterson (1991), that S. ludovici and S. erythromos form sister pairs with S. oporaphilum and S. bogotensis respectively. Two new taxa are Marginal means (Bonferroni) for RW I S. perla vs S. lilium <0.001 S . perla vs. S. luisi <0.001 S . perla vs. S. tildae <0.001 Marginal means (Bonferroni) for RW II S. perla vs S. lilium 0.08

S. perla vs. S. luisi 1

S. perla vs. S. tildae 1

MANOVA FOR RW I AND ACM p -value Effect size (ƛ2) Equality of covariance Box's M=31.72, F=1.36, df=21/2568.61 0.13

Equality of error variances RW I - Levene's F=2.09, df=7/199 0.05 ACM - Levene's F=2.03, df=7/199 0.05

Joint cetroids (MANOVA) Species - Pillai's trace=0.42, F=17.71, df=6/398 <0.001 0.21 Sex - Pillai's trace=0.01, F=0.94, df=2/198 0.39 0.01 Species X Sex - Pillai's trace=0.03, F=1.09, df=6/398 0.37 0.02 also mentioned in Iudica’s hypothesis, corresponding to the S. sp. A and S. sp. B of Albuja (1999). Finally, Villalobos and Valerio (2002) combined the morphological data previously published by Owen (1987) and Pacheco and Patterson (1991) ( Fig. 8 D). They presented a consensus tree that, for the first time, characterized a monophyletic group formed by Sturnira lilium , S. luisi , S. tildae , S. aratathomasi , and S. thomasi . They also resolved the relationships for the other species, with S. magna at the base of the Sturnira subgenus, and a ladder-like phylogenetic relationship among the remaining species, with S. mordax and S. ludovici at the base of this subgroup. In spite of being the first study to propose a fully resolved evolutionary hypothesis for the basal groups of the genus, Villalobos and Valerio (2002) did not include the rest of the taxa recognized as valid by Iudica (2000). Unfortunately, the only phylogenetic hypothesis to receive indexes of topological support was Iudica’s ( Fig. 8 C).

Within this context, our hypothesis maintains the position of both Sturnira bidens and S. nana as basal. Yet, we suggest that S. nana shares a more common recent ancestor with all other species except for S. bidens , being this last species at the deepest node. There is considerable substructure in the taxa named as S. lilium , with at least two major branches, both of Central and South American origin. Also, S. ludovici shows large substructure, with one branch closer to S. oporaphilum from Peru and other closer to S. hondurensis from Honduras. S. magna , S. erythromos and S. bogotensis seem to share common ancestry. These three last taxa form a sister branch with S. tildae , S. mordax , S. koopmanhilli and S. perla , all four in a politomy. Overall there is strong support for most nodes, except for relatively large uncertainty at two basal nodes holding most of the highland (e.g. S. erythromos and S. ludovici ) and lowland species (e.g. S. lilium and S. luisi ), with posterior probabilities of 0.51 and 0.64 respectively.

A detailed assessment of these hypotheses is beyond the scope of this study. For now, it may be safe to note that S. perla maintains relatively sound genetic distances to other species; having, in consequence a large branch in our proposed phylogeny. S. perla may have closer affinities to highland species (e.g. S. ludovici and S. erythromos ) rather than to lowland taxa (e.g. S. luisi and S. lilium ). In this case, Iudica (2000) was unable to find support for any sound relationship of S. perla (S. sp. B) to other taxa.

Conservation status. All 14 specimens known for this species come from a highly devastated area, suffering from an accelerated rate of deforestation ( Brooks et al. 2002; Rival 2003; Fig. 3 ), amidst a human population affected from increasingly levels of social unrest and poverty ( Sierra & Stallings 1998). The most recent collection records for Sturnira perla are from over a decade ago. The remaining Choco forest in the Neotropics represents 24% of the original landcover and only 18% in Ecuador ( Sierra et al. 2002). Rates of deforestation in western Ecuador are comparatively high, ranging from 2% to 4% per year ( Brooks et al. 2002; Sierra & Stallings 1998), one of the highest rates of loss in Latin America ( Rudel 2000). Seven of the fourteen specimens known for S. perla were collected from an isolated patch consisting of 226 ha of primary forest, known as “Bosque Protector la Perla ” ( Botero 2004), and surrounded by vast expanses of monocultures consisting mostly of grazing lands, oil palms and banana trees. The Choco is well known for its biological richness ( Gentry 1993), and high levels of vertebrate endemism ( Mittermeier et al. 1999; Myers et al. 2000). According to Chapman (1917:106), the fauna living in the Choco is highly unique relative to other regions in South America. Some suggest that the Choco contains the highest number of plant species ever to have been reported for 0.1 ha, being perhaps the region with the highest richness of plant species on Earth ( Galeano et al. 1998). However, this noteworthy but unknown species of mammal may be experiencing a tragedy. The same kind of tragedy that for Susy Sheppard, the late protector and owner of “La Perla ”, was “to see that wonderful forest, that covered hills and valleys, converted into grasslands” ( Botero 2004).

Ecology. Considering that skull shape is a well known indicator of feeding behavior in bats (e.g. Freeman, 1981; Muchhala & Jarrín-V. 2002; Dumont and Herrel, 2003), the distinct shape of the skull in Sturnira perla , relative to the highly overlapped distribution that other lowland species have in morphometric space, suggests that this species may also be remarkably divergent in its ecological habits, especially those immediately related to feeding behavior. The ecological distinctiveness of S. perla , in direct relationship to its remarkable skull shape, remains as a hypothesis. Further studies are necessary in this sense, especially given the accelerated rate of deforestation in Northwestern Ecuador (i.e. the Southernmost Choco).

Figures

FIGURE 2. Individual aspects of the skull and mandible for Sturnira perla and S. luisi in dorsal, ventral and lateral views. The skull of the former species has an overall rounded shape relative to the latter.

FIGURE 3. The five localities from where Sturnira perla is currently known, represented as dark or white spots correspondingly to each map. A considerable expanse of the original Chocoan forest has been replaced by agriculture. The depicted estimate of remaining vegetation dates to 1996, in which the amount of altered vegetation must have increased considerably since then. Numbers related to each location in the map are included in parenthesis next to the corresponding locality for S. perla in Appendix 1.

FIGURE 4. Frequency distribution for the characters FA and GLS. The delimitation of distinct groups is not possible along both distributions. Species included in both histograms are Sturnira. perla, S. luisi, S. lilium and S. tildae.

FIGURE 5. Aspects of the morphometric space for Sturnira. A) Plot of the first two principal components, based on the covariance of 27 interlandmark characters. B) Plot of the second and third principal components extracted from the covariation of interlandmark characters. C) Plot of the first two relative warps of geometric variation of the skull. D) Plot of the ACM and RW I. The percentage of explained variation by each PC or RW is in parenthesis. Despite the large overlap in other groups, Sturnira perla, highlighted by a convex hull, appears as a remarkably distinctive group. The arrows indicate the position of the estimated shape configurations depicted as a and b. The morphometric space around S. perla is for skulls with expanded braincases, wide and blunt rostrums and a contracted shape around the zygomatic area (a). An opposite pattern of shape variation is shown for the area around the selected sample that represents S. lilium (b).

FIGURE 6. Mean distances for the Kimura 2 - parameter model (K 80) among species in Sturnira. The largest difference belongs to S. bidens from Peru (CAI 208). S. perla maintains average distances to other species, but sufficiently large to support its individuality as an evolutionary lineage according to Bradley and Baker (2001).

FIGURE 8. Past phylogenetic hypotheses for Sturnira: A) Owen (1987), B) Pacheco and Patterson (1991), C) Iudica (2000) and D) Villalobos and Valerio (2002). The four hypotheses depicted here are exact representations of the final consensus cladograms in the original publications. A, B and D lack support indexes for branch topology.

FIGURE 7. A phylogenetic hypotheses inferred from Bayesian posterior probabilities with the GTR + I + Γ substitution model. This hypothesis is a consensus of agreement in bipartitions which are higher than 50 % of all trees estimated (136500 trees). Support values at nodes are posterior probabilities. S. perla seems to be more related to highland taxa; a perspective that runs contrary to its general morphology (i. e. shape of cusps in lower molars) and previous phylogenetic hypotheses (Iudica, 2000).

Tables

TABLE 1. Descriptive statistics for interlandmark characters and ACM. Total sample size is n = 279 except for the ACM with

Angle of curvature of the zygomatich arch*	Sturnira perla 146.5 [144.31–148.69] (140–151) 3.45	S. lilium 158.95 [157.79–160.11] (149–173) 5.92	S. luisi 158.34 [157.07–159.61] (149–174) 5.6	S. tildae 158.6 [156.07–161.13] (152–166) 4.56
Forearm length	43.1 [42.71–43.5] (42.13–44.38) 0.62	42.63 [42.41–42.86] (38.43–44.99) 1.39	42.82 [42.51–43.12] (37.26–47.44) 1.58	46.57 [46.05–47.09] (45.01–48.42) 1.05
Third metacarpal length	43.5 [42.78–44.22] (41.98–46.35) 1.14	42.15 [41.88–42.41] (37.12–46.88) 1.59	42.47 [42.15–42.79] (38.53–48.16) 1.66	45.92 [44.97–46.87] (42.22–49.1) 1.91
Fourth metacarpal length	42.53 [42.17–42.89 (41.31–43.42) 0.57	41.68 [41.43–41.93] (36.98–46.22) 1.51	41.89 [41.58–42.2] (38.26–47.64) 1.62	45.51 [44.55–46.46 (41.37–48.23) 1.92
Fifth metacarpal length	43.64 [43.15–44.12] (41.98–44.76) 0.77	42.96 [42.71–43.22] (38.6–47.9) 1.55	43.07 [42.73–43.41] (39.37–49.0) 1.76	46.85 [45.82–47.87] (42.52–50.24) 2.06
Tibia length	17.63 [16.92–18.34] (15.1–18.56) 1.12	17.17 [16.94–17.41] (12.47–19.94) 1.43	17.24 [16.99–17.49] (12.58–20.07) 1.3	19.35 [18.81–19.88] (17.41–21.19) 1.08
Hind foot length	13.32 [12.81–13.82] (11.84–14.58) 0.8	13.36 [13.19–13.53] (11.1–16.48) 1.04	12.92 [12.68–13.15] (10.54–16.5) 1.21	13.62 [13.12–14.12] (11.76–15.38) 1.0
Height of the skull	11.17 [10.98–11.36] (10.72–11.62) 0.29	11.16 [11.08–11.25] (9.15–13.04) 0.51	11.25 [11.16–11.34] (10.1–12.34) 0.48	11.37 [11.19–11.54] (10.94–12.17) 0.35
Greatest length of the skull	22.71 [22.5–22.92] (22.1–23.12) 0.11	23.15 [23.04–23.26] (21.35–24.65) 0.67	23.28 [23.13–23.43] (21.37–25.05) 0.79	23.97 [23.64–24.3] (22.99–25.54) 0.67
Occipital condyles – incissors length	20.39 [20.22–20.56] (19.77–20.78) 0.27	20.89 [20.78–21.0] (19.1–23.27) 0.69	20.97 [20.83–21.12] (19.09–22.96) 0.76	21.82 [21.49–22.15] (20.5–23.3) 0.66
Auditory bula–incisors length	17.79 [17.64–17.94] (17.37–18.04) 0.23	18.26 [18.16–18.36] (16.68–20.92) 0.62	18.32 [18.2–18.45] (16.61–20.06) 0.64	18.99 [18.69–19.29] (17.73–20.25) 0.61
Mastoid breath	11.82 [11.69–11.94] (11.34–12.15) 0.2	12.03 [11.98–12.09] (10.92–12.99) 0.35	12.16 [12.08–12.24] (11.21–13.24) 0.4	12.64 [12.4–12.88] (11.7–13.52) 0.48
Zigomatic breath	14.51 [14.31–14.71] (13.79–14.85) 0.31	13.72 [13.66–13.78] (12.49–14.82) 0.38	13.79 [13.69–13.89] (11.95–14.95) 0.51	14.17 [13.93–14.42] (13.35–15.24) 0.49
Postorbital constriction	5.78 [5.71–5.85] (5.62– 5.94) 0.11	5.83 [5.8–5.87] (5.22– 6.32) 0.22	5.9 [5.85–5.94] (5.38– 6.58) 0.24	6.12 [5.92–6.31] (5.47–7.25) 0.39
Braincase length	7.67 [7.5–7.84] (7.07– 8.09) 0.27	8.31 [8.24–8.37] (7.42– 9.46) 0.39	8.4 [8.32–8.47] (7.33– 9.57) 0.38	8.79 [8.6–8.98] (8.29– 9.69) 0.38
Braincase width	10.34 [10.23–10.45] (10.07–10.63) 0.17	10.52 [10.47–10.57] (9.8–11.73) 0.31	10.53 [10.47–10.6] (9.68–11.27) 0.34	10.75 [10.57–10.92] (9.92–11.34) 0.36
Breadth accross fora- men magnum	6.43 [6.31–6.55] (6.12– 6.63) 0.19	6.36 [6.32–6.41] (5.66– 6.93) 0.26	6.4 [6.35–6.45] (5.49– 7.04) 0.27	6.77 [6.62–6.92] (6.09–7.26) 0.3
Nasal breadth	3.26 [3.19–3.33] (3.08– 3.41) 0.11	3.21 [3.19–3.23] (2.85– 3.58) 0.15	3.22 [3.19–3.25] (2.73– 3.61) 0.16	3.43 [3.36–3.51] (3.19–3.7) 0.15
Palatal length	10.11 [9.97–10.25] (9.84–10.59) 0.22	10.15 [10.08–10.22] (8.58–11.18) 0.44	10.18 [10.09–10.26] (8.79–11.15) 0.45	10.55 [10.34–10.76] (9.9–11.53) 0.42
Palatal breadth	8.23 [8.14–8.32] (7.91– 8.46) 0.14	8.2 [8.16–8.24] (7.43– 8.91) 0.25	8.2 [8.14–8.26] (7.42– 8.84) 0.3	8.27 [8.15–8.38] (7.84–8.67) 0.23

TABLE 3. Results of a MANOVA for linear characters among species and sex. The MANOVA includes ACM and PC I – PC V as response variables. The results of the Bonferroni tests between marginal means for the factor species are shown only for those with p <0.05 along ACM, PC I and PC II. Differences are concentrated around Sturnira perla along PC II and ACM, and around S. tildae along PC I. Sample size is Sturnira lilium = 103, S. luisi = 76, S. perla = 12 and S. tildae = 15.

Equality of error variances	ACM - Levene's F=2.26, df=7/198	0.03
	PC I - Levene's F=2.53, df=7/198	0.02
	PC II - Levene's F=1.72, df=7/198	0.11
	PC III - Levene's F=0.48, df=7/198	0.85
	PC IV - Levene's F=0.84, df=7/198	0.55
	PC V - Levene's F=1.15, df=7/198	0.33

TABLE 4. Results of a MANOVA for the first two relative warps (RW I-RW II) and a MANOVA for ACM and RW I among species and sex. The results of the Bonferroni tests between marginal means for the factor species are shown only for those with p <0.05 along ACM, RW I and RW II. The sole responsible for the observed differences is Sturnira perla on the three response variables. Sample size is Sturnira lilium = 103, S. luisi = 76, S. perla = 12 and S. tildae = 16.

MANOVA FOR RW I AND RW II Equality of covariance	p -value Box's M=27.26, F=1.17, df=21/2551.49 0.27	Effect size (ƛ2)
Equality of error variances	RW I - Levene's F=2.1, df=7/208 0.05 RW II - Levene's F=1.67, df=7/208 0.12
Joint centroids (MANOVA)	Species - Pillai's trace=0.43, F=18.91, df=6/416 <0.001 Sex - Pillai's trace=0.02, F=1.53, df=2/207 0.22 Species X Sex - Pillai's trace=0.04, F=1.22, df=6/416 0.29	0.21 0.02 0.02
Centroids (ANOVA)	RW I on Species - F=14.93 <0.001 RW II on Species - F=15.06 0.003 RW I on Sex - F=2.58 0.41 RW II on Sex - F=9.7 <0.11	0.37 0.06 <0.01 0.01